TCM Arithmetic 1: Decimal System and Counting Rods

By: Tao Steven Zheng (郑涛)

Chinese Numerals and the Decimal System

The Chinese adopted a decimal (base-ten) numeral system as early as the fourteenth century BC, during the Shang dynasty (c.1600 – 1046 BC). This is evidenced by the earliest literary inscriptions, called jiaguwen (甲骨文, “oracle bone script”), which were etched on durable media such as tortoise plastrons and the shoulder bones of cattle. Many of the jiaguwen documents recorded special events, oracle queries, as well as astronomical observations. Fortunately, the Shang scribes left behind records that include numerals (Figure 1).

Figure 1. Jiaguwen numerals from the Shang dynasty (c. 1600 - 1046 BC)

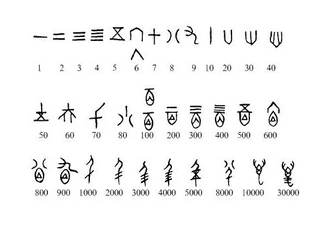
There were originally thirteen distinct symbols for the numbers one to nine, ten, hundred, thousand, and myriad (ten thousand). For example, the number 51238 would be literally expressed as five-myriad one-thousand two-hundred three-ten eight 五萬一千二百三十八 (wu wan yi qian er bai san shi ba). Symbols for larger powers of ten and zero would appear in later dynasties. Table 1 shows the original Chinese numerals and their equivalent Hindu-Arabic numeral.

Table 1. Chinese numerals

Number	Hindu-Arabic Numerals
一 yi	1
二 er	2
三 san	3
四 si	4
五 wu	5
六 liu	6
七 qi	7
八 ba	8
九 jiu	9
十 shi	10
百 bai	100
千 qian	1000
萬 wan	10000

Counting Rods

Figure 2. Ivory counting rods

By the Spring-Autumn (771 – 476 BC) and Warring States period (476 – 221 BC), Chinese arithmeticians have transitioned to the use of counting rods for representing numbers and performing calculations. There were many names for counting rods: chou suan (籌算), chou ce (籌策), suan ce (算策), and suan zi (算子). Each name suggests that they were used for calculation and planning. The counting rods were made of a variety of materials, from wood and bamboo to more expensive materials such as bone, bronze, and ivory (Figure 2).

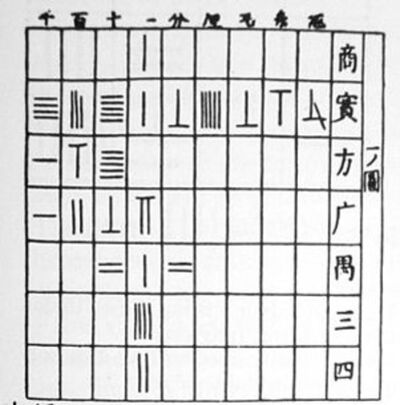
Figure 3. Counting board with counting rods

The counting rods were arranged on a flat surface such as a table or the ground; more elaborate surfaces included counting boards that included marked square cells to demarcate rows and columns (Figure 3). According to the Sunzi Suanjing (孙子算经, Sunzi’s Mathematical Manual), rods representing the digits of even powers of ten are placed vertically, while rods representing digits of odd powers of ten are placed horizontally.

凡算之法，先識其位，一從十橫，百立千僵，千十相望，萬百相當。

For the method of computation, one must first know the positions [of the rod numerals]. The units are vertical and the tens horizontal, the hundreds stand and the thousands prostrate; thousands and tens look alike, and so do ten thousands and hundreds.

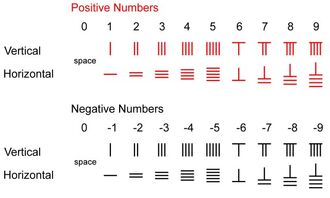
This rule was adopted to avoid confusion when performing arithmetic calculations. Any place value where zero would appear were simply left blank; this was called kong (空, literally “empty” or “void”). The concept and application of negative numbers first arose in ancient China. As early the Han dynasty (206 BC – 220 AD), coloured rods were used to differentiate positive numbers from negative numbers (Figure 4). Alternatively, a single rod set diagonally across the rods representing a given number could also signify a negative number. According to Liu Hui’s commentary of the Jiuzhang Suanshu (九章算术, Nine Chapters on the Mathematical Art):

今兩筭（算）得失相反，要令正負以名之。正算赤，負算黑。否則以邪（斜）正為異。

Two kinds of counting rods represent the mutual opposites of gains and losses. Let them be named positive and negative.  The positive rods are red, and the negative rods are black. Otherwise, they can be differentiated by being placed slanted or upright.

Figure 4. Chinese rod numbers

Chinese counting rods were not only used for performing arithmetic. By the Han dynasty, counting rods were used to represent the coefficients of simultaneous linear equations, much like how matrices are used in modern mathematics. During the Song dynasty, Chinese mathematicians went as far to developing a notation for representing polynomial equations.

Arithmetic with Counting Rods

Figure 5. Multiplication table from the Tsinghua Bamboo Strips

Counting rods served as a tool for representing numbers and carrying out the steps of computation, which enabled the arithmetician to perform calculations of arbitrary complexity with relative ease. Systematic and efficient algorithms for addition, subtraction, multiplication, and division were developed to perform such tasks. More remarkably, algorithms for calculating square roots and cube roots were developed by the Han dynasty (the square root and cube root algorithms will be examined in more detail in the algebra section due to its connection to solving quadratic, cubic, and higher-degree polynomial equations). For multiplication, division, and the extraction of roots, the ancient Chinese arithmeticians are required to memorize the multiplication table. In fact, the world's oldest decimal multiplication table was excavated in China, and is radiocarbon dated to roughly 305 BC. The multiplication table now belongs to a collection of Chinese texts called the Tsinghua Bamboo Strips (清華簡 qing hua jian). The multiplication table was written in ink on bamboo strips and its content includes the 9 x 9 multiplication table, products with half-integers, and select products up to 90 squared.

Problem Study 1: Multiplication with Counting Rods

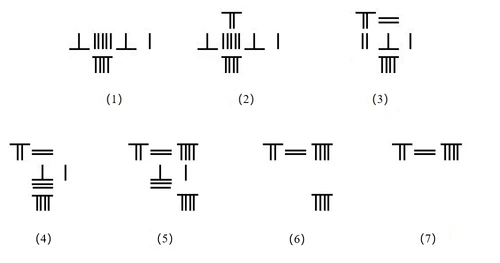
The following problem from the Sunzi Suanjing (Chapter 1, Problem 16) demonstrates how ancient Chinese arithmeticians performed multiplication and addition. When performing multiplication, the two multiplicands are set in the upper position (上位 shang wei) and lower position (下位 xia wei); the product rests in the middle position (中位 zhong wei).

There are three positions when implementing rod numerals to perform multiplication and division: upper position (上位 shang wei), middle position (中位 zhong wei), and lower position (下位 xia wei). When multiplying two numbers, rods set in the upper position represent the multiplier; rods placed in the lower position represent the multiplicand; lastly, rods placed in the middle position represent the product. Figure 5 illustrates the multiplication algorithm using counting rods.

九九八十一，自相乘，得幾何？ 答曰：六千五百六十一。 術曰：重置其位，以上八呼下八，八八六十四，即下六千四百於中位。以上八呼下一，一八如八，即於中位下八十。退下位一等，收上位八十。以上位一呼下八，一八如八，即於中位下八十。以上一呼下一，一一如一，即於中位下一。上下位俱收，中位即得六千五百六十一。

Nine times nine makes 81. What is the amount when this is multiplied by itself? Answer: 6561. Method:  Set up the two positions [upper position and lower position]. The 8 in the upper position calls the 8 in the lower position, eight times eight makes 64, so put down 6400 in the middle position. The upper 8 calls the lower 1: one times eight is 8, so put down 80 in the middle position. Shift the lower numeral one place [to the right] and put away the 80 in the upper position. The upper 1 calls the lower 8: one times eight is 8, so put down 80 in the middle position. The upper 1 calls the lower 1: one times one is 1, so put down 1 in the middle position. Remove the rod numerals in the upper and lower positions leaving 6561 in the middle position.

(1) Set up the two positions [upper position and lower position].

	千	百	十	一
上位			8	1
中位
下位		8	1

(2) The 8 in the upper position calls the 8 in the lower position, eight times eight makes 64, so put down 6400 in the middle position.

	千	百	十	一
上位			8	1
中位	6	4
下位		8	1

(3) The upper 8 calls the lower 1: one times eight is 8, so put down 80 in the middle position.

	千	百	十	一
上位			8	1
中位	6	4	8
下位		8	1

(4) Shift the lower numeral one place [to the right] and put away the 80 in the upper position.

	千	百	十	一
上位				1
中位	6	4	8
下位			8	1

(5) The upper 1 calls the lower 8: one times eight is 8, so put down 80 in the middle position.

	千	百	十	一
上位				1
中位	6	4	8 + 8	0
下位			8	1

	千	百	十	一
上位				1
中位	6	5	6
下位			8	1

(6) The upper 1 calls the lower 1: one times one is 1, so put down 1 in the middle position.

	千	百	十	一
上位				1
中位	6	5	6	1
下位			8	1

(7) Remove the numerals in the upper and lower positions leaving 6561 in the middle position.

	千	百	十	一
上位
中位	6	5	6	1
下位

Figure 5. Multiplication with counting rods

Problem Study 2: Division with Counting Rods

The following problem is from the Sunzi Suanjing (Chapter 1, Problem 17). Similar to multiplication, three positions are used; however, this time the middle position represents the dividend (實 shi), the lower position represents the divisor (法 fa), and the upper position represents the quotient (商 shang). Figure 6 illustrates the division algorithm using counting rods.

六千五百六十一，九人分之，問：人得幾何？ 答曰：七百二十九。 術曰：先置六千五百六十一於中位，為實。下列九人為法。上位置七百，以上七呼下九，七九六十三，即除中位六千三百。退下位一等，即上位置二十。以上二呼下九，二九十八，即除中位一百八十。又更退下位一等，即上位更置九，即以上九呼下九，九九八十一，即除中位八十一。中位並儘，收下位。上位所得即人之所得。自八八六十四至一一如一，並準此。

Divide 6561 evenly among 9 people. Question: How much does each person receive? Answer: 729. Method: First set 6561 in the middle position to be the dividend (实 shi ). Below it, set 9 persons to be the divisor (法 fa). Put down 700 in the upper position. The upper 7 calls the lower 9: seven nines are 63, so remove 6,300 from the numeral in the middle position. Shift the numeral in the lower position one place [to the right] and put down 20 in the upper position. The upper 2 calls the lower 9: two nines are 18, so remove 180 from the numeral in the middle position. Once again shift the numeral in the lower position one place [to the right], and put down 9 in the upper position. The upper 9 calls the lower 9: nine nines are 81, so remove 81 from the numeral in the middle position. There is now no numeral in the middle position. Put away the numeral in the lower position. The result in the upper position is what each person gets.

(1) First set 6561 in the middle position to be the dividend (實 shi). Below it, set 9 persons to be the divisor (法 fa).

	千	百	十	一
上位（商）
中位（實）	6	5	8	1
下位（法）		9

(2) Put down 700 in the upper position. The upper 7 calls the lower 9: seven nines are 63, so remove 6,300 from the numeral in the middle position.

	千	百	十	一
上位（商）		7
中位（實）	6	5	8	1
下位（法）		9

(3) Shift the numeral in the lower position one place [to the right] and put down 20 in the upper position.

	千	百	十	一
上位（商）		7	2
中位（實）		2	6	1
下位（法）			9

(4) The upper 2 calls the lower 9: two nines are 18, so remove 180 from the numeral in the middle position.

	千	百	十	一
上位（商）		7	2
中位（實）			8	1
下位（法）			9

(5) Once again shift the numeral in the lower position one place [to the right], and put down 9 in the upper position.

	千	百	十	一
上位（商）		7	2	9
中位（實）			8	1
下位（法）				9

(6) The upper 9 calls the lower 9: nine nines are 81, so remove 81 from the numeral in the middle position.

	千	百	十	一
上位（商）		7	2	9
中位（實）
下位（法）				9

(7) There is now no numeral in the middle position. Put away the numeral in the lower position. The result in the upper position is what each person gets.

	千	百	十	一
上位（商）		7	2	9
中位（實）
下位（法）

Figure 6. Division with rod numerals

Legacy of Counting Rods

For much of Chinese history, especially from the Han to the Yuan dynasties (206 BC - 1368 AD), counting rods played an important role in the development of Chinese mathematics. The Chinese counting rods alongside gradually spread throughout the Sinosphere (Korea, Japan, and Vietnam). After the Yuan dynasty, counting rods fell into disuse in China, and were replaced by the abacus. Mathematical texts written in the Ming dynasty (1368 – 1644 AD) introduced new computation methods adapted to the abacus.  This “mechanization” of computation coincided with a decline in the theoretical development of traditional Chinese mathematics. However, during the Edo period of Japan (1603 – 1867 AD), notable Japanese mathematicians continued the use of counting rods in their research (Figure 7). This blossoming tradition, called wasan (和算, “Japanese mathematics”), extended the developments of Chinese mathematics to new heights, rivalling the increasingly advanced European mathematics of the 17th and 18th centuries.

Figure 7. Japanese depiction of a mathematician manipulating counting rods on a counting board

Aftermath: The Chinese Abacus

Figure 8. Chinese abacus

The suan pan (算盤, 'computation tray'), or Chinese abacus, is a calculation device that consists of a rectangular frame lined with eight or more bamboo rods and sliding beads suspended on each rod. A wooden divider separates the abacus into two tiers. Its characteristic design with two beads on each rod in the top tier, and five beads on each rod in the bottom tier has become a de-facto icon of all abacuses. The exact origins of the Chinese abacus is obscure, with the earliest surviving description of a calculation method using beads in the Suanshu Jiyi (算數記遺, Notes on Traditions of Arithmetic Methods). The Suanshu Jiyi is a text written around 190 AD by the astronomer and mathematician Xu Yue (徐岳, c. 180 - 220 AD). Xu Yue calls this calculation method 珠算 (zhu suan, 'bead arithmetic'), a term still used today for the art of calculation with the abacus, not the device itself.

刻板為三分，其上下二分以停遊珠，中間一分以定算位，位各五珠，上一珠與下四珠色別，其上別色之珠當五，其下四珠，珠各當一。

A grooved board is split into three sections, with moveable beads placed in the top and bottom sections, and a middle section for setting the positions of computation. Each position [column] contains five beads, with one bead in the upper section and four beads of a different colour in the bottom section: the top beads of one colour are worth five; the four bottom beads each being worth one.

Figure 9. Possible depiction of a suan pan in the Qingming Shanghe Tu.

It is uncertain whether or not the grooved abacus with rolling beads described in the Suanshu Jiyi was commonly used during the Han dynasty, for most historical accounts suggest that counting rods had served as chief computation tool prior to the 16th century. What may be the earliest depiction of the suan pan can be found in the Qingming Shanghe Tu (清明上河圖, Along the River During Qing Ming Festival), a painted scroll by Zhang Zeduan (1085 – 1145 AD) during the Song Dynasty (960 - 1279 AD). The painting shows what appears to be a suan pan on the counter of an apothecary. However, despite the the Song dynasty being reputed to have a thriving market economy, there is no textual evidence that suggests the commercial use of the suan pan at the time. What is certain is that the suan pan entered into common use in China during the Ming dynasty. By this time, the Chinese abacus had already evolved to what is recognizably the modern suan pan, and numerous manuals on how to operate the suan pan were already in circulation. Knowledge and use of the suan pan quickly made its way to Korea, Japan, and Vietnam, making it the computational tool of choice by merchants, accountants, and clerks in East Asia.

Additional Problems

Problem 1

Read the following passage. Illustrate the multiplication process using rod arithmetic (Arabic numbers may be used).

《孙子算经·卷下4》 今有佛书凡二十九章，章六十三字。问字几何？ 答曰：一千八百二十七。 术曰：置二十九章，以六十三字乘之，即得。

Sunzi Suanjing (Juanxia 4) Suppose there is a Buddhist sutra with 29 chapters. Each chapter contains 63 words. How many words are there? Answer: 1827. Method: Set 29 chapters and multiply by 63 characters to obtain the answer.

Solution

(1)

	千	百	十	一
上位			2	9
中位
下位		6	3

(2)

	千	百	十	一
上位			2	9
中位	1	2	0	0
下位		6	3

(3)

	千	百	十	一
上位			2	9
中位	1	2	6	0
下位		6	3

(4)

	千	百	十	一
上位				9
中位	1	2	5	0
下位			6	3

(5)

	千	百	十	一
上位				9
中位	1	8	0	0
下位			6	3

(6)

	千	百	十	一
上位				9
中位	1	8	2	7
下位				3

(7)

	千	百	十	一
上位
中位	1	8	2	7
下位